There are many statistical concepts that come in handy, when handling large data sets. In this article, the calculation technique for relative standard deviation is presented in brief.
One of the most important parts of any experiment is the mathematical analysis of its results. A branch of mathematics which is completely devoted to the study of data and its analysis is statistics. There are several statistical constructs, which are regularly employed when analyzing experimental data.
Calculation Technique
Let me briefly explain how to calculate the mean and standard deviation for any data set.
The mean of any data set is obtained by summing up all its readings and dividing by their total number. For an array of data points X, with values ranging from x1, x2, … xN, the mean will be:
Mean for the Array X = XMean = (Σn=1N xn)/N
The standard deviation of a data set, gives you an idea about how precise the experimental results are and how much they deviate from the mean. A high value points towards a highly imprecise set of results, while a low value indicates that the results are closer to the mean value. Here is the formula:
Standard Deviation (σ) = √[{Σn=1N (xn – XMean)2}/{N – 1}]
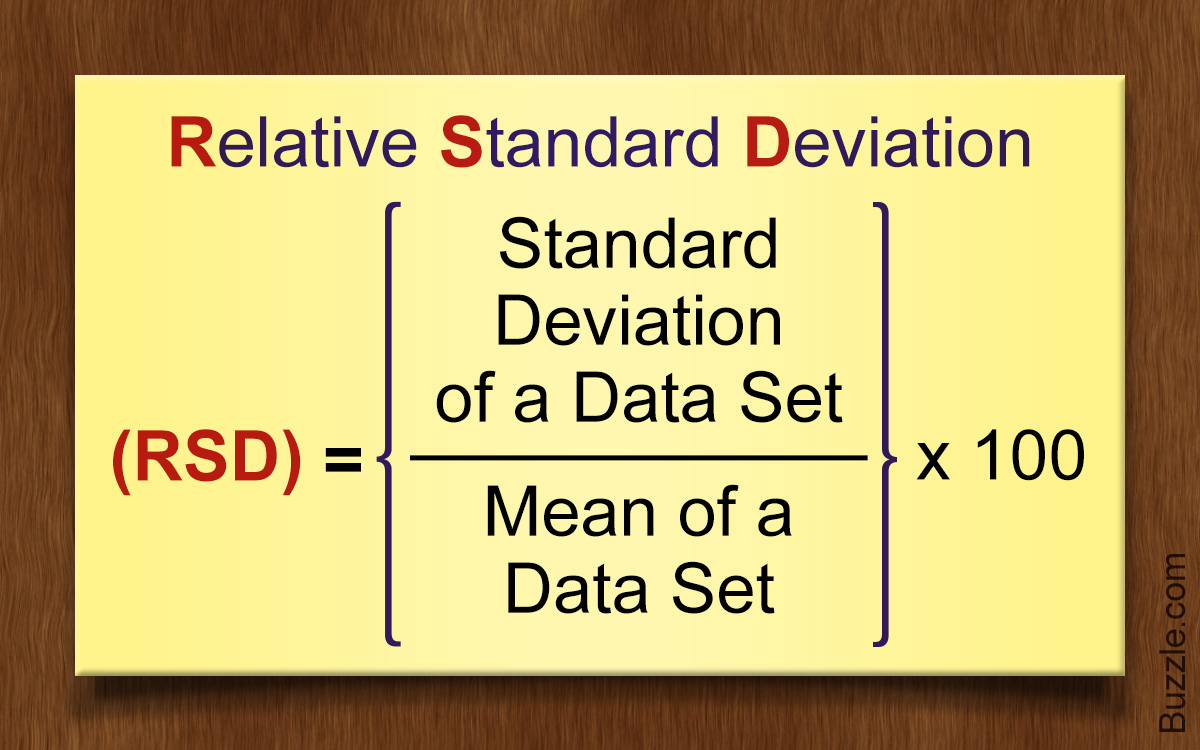
Relative standard deviation is the ratio of standard deviation of a data set, to its mean, which is then multiplied by 100. It is used to compare the error in different data sets, with different mean values. As the units of mean and standard deviation are same, this ratio is a pure number, with no associated units.
Equation
This ratio is also known as percent standard deviation, as after all, it is a percentage. Here is the formula used for calculation.
Relative Standard Deviation (RSD) = (Standard Deviation of a Data Set/Mean of a Data Set) x 100
Calculation Procedure
Here is the procedure:
- First calculate the mean of the data set, by summing up all values and dividing it by their total quantity.
- Once you get the mean, the next part is to calculate standard deviation.
- To do so, subtract all the data readings from the mean value, square the difference for each value and calculate the sum of the squares for all values.
- Then, using the formula listed above, calculate it.
- Divide the standard deviation by the mean of the data set and multiply it by 100, to get the percent relative standard deviation.
If you are a student of chemistry or physics, you are bound to employ this calculation method when analyzing experimental data, generated in the lab.