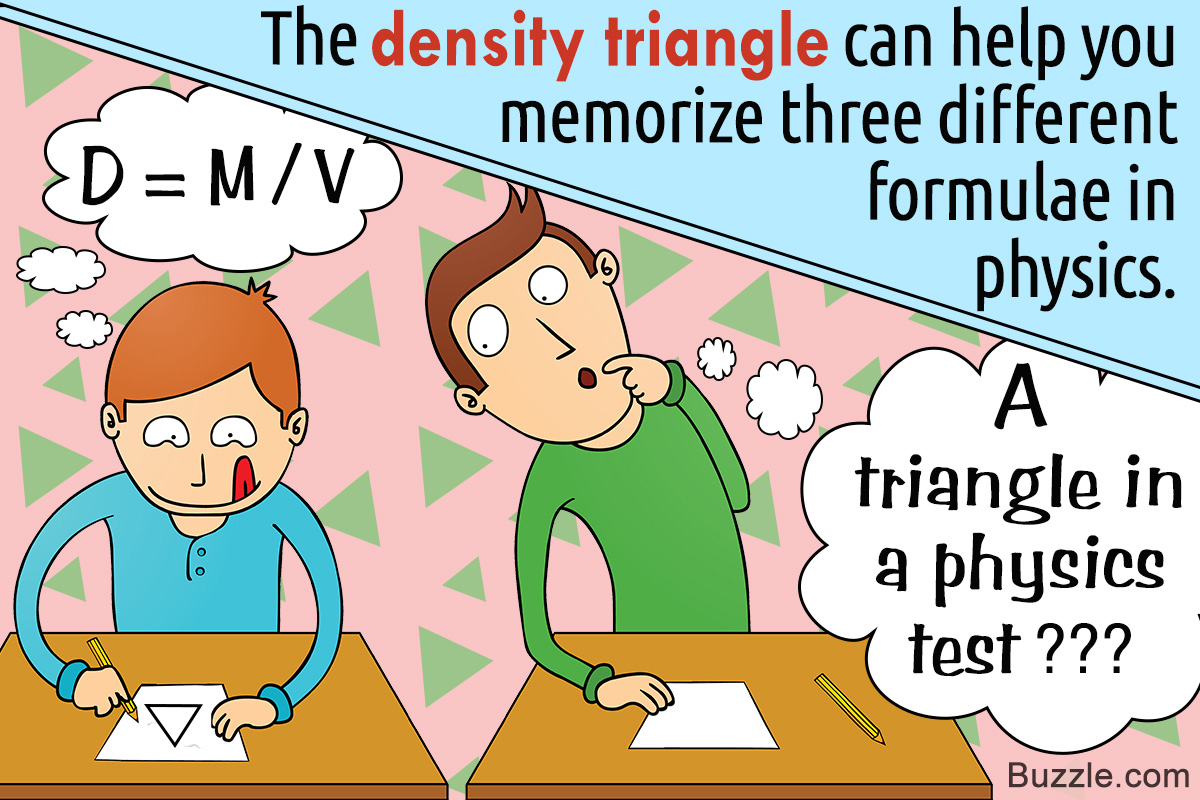
The density triangle in physics is a handy memory tool used by students to memorize three different but interrelated formulae. In this ScienceStruck post, we show you how to use the density triangle to make your life in the world of physics a tad bit easier.
Did you know?
The density triangle isn’t the only memory tool used by physicists. There is another triangle, known as the V-I-R triangle, which can be used in a similar manner. It can be obtained simply by replacing D, M, and V in the density triangle, by I, V, and R, respectively. Using the same multiplication and division rules, the V-I-R triangle can help memorize the three formulas that comprise Ohm’s law.
The world of physics is a frightening place. Enter in through its gates, and you are greeted by the thunderous howls and shrieks of several complicated concepts, that shake your very soul. As you cover your ears and run further ahead, your path is blocked by numerous long serpentine mathematical equations, just waiting to coil around your head and devour that last bit of hope left within your heart.
Did I scare you? I hope not. Trust me, I am not here to spell doom. I am here to tell you that though learning physics may seem like a daunting task, there are tricks which you can use to smoothly navigate through its scary jungles. The density triangle is one such neat memory trick that can help you tackle, not one, not two, but three physical concepts, in one fell swoop. So, if I have sparked your interest, then without further ado, let’s get to know more about this density triangle.
Need for the Density Triangle
Every physical object is made up of matter. As such, it has three measurable quantities associated with it, namely – density, mass, and volume. The following are the definitions for each.
Mass
The total amount of matter present within an object or a body is known as its mass. Its S.I. unit of measurement is kg. Note that mass is different from weight, which represents the gravitational force of attraction on an object. For example, a person will weigh less on the moon due to its weaker gravity as compared to Earth, but would have the same mass in both the places.
Volume
The overall space occupied by a body in a 3D space is known as its volume. Volume, thus, represents the body’s expanse in all three dimensions, represented by its length, width, and height. The S.I. unit for each of these is meter (m), and since volume is a product of the three, its unit is m3.
Density
The density of an object is the measurement of its compactness. Basically, it tells you how closely crammed matter is within that object. Mathematically, it is defined as the mass per unit volume of a body.
From these definitions, it is clear that density is a function of both the mass as well as the volume of an object. Its mathematical equation comprises both these quantities. By rearranging the terms of this equation, two more equations can be derived – one to calculate the mass and another to calculate the volume. So effectively, if you can remember any one of them, you can easily find out the remaining two. However, the downside to this is that, if you get the first one wrong, then the remaining two shall also go wrong. To avoid such a situation, the density triangle was conceived.
How Does the Density Triangle Work
An old Chinese proverb – I hear and I forget, I see and I remember, I do and I understand. The density triangle is in keeping with the first part of this proverb. It gives you a visual representation, which helps you easily remember the three density triangle formula or equations. The following is what a density triangle looks like.

The M on top represents the mass of a body, while the D and V at the bottom represent its density and volume, respectively. Using it, three density triangle equations can be derived, one each for calculating the mass, density, and volume of an object. Hence, it is also sometimes called the mass volume density triangle.
To obtain these three equations, all you need to do is memorize this one triangle, and then use the procedure described below.
Formula for Density

To get the formula for density, simply cover the ‘D’ part with your fingers. Now what remains is M and V. Notice that M is above and V is below, separated by a horizontal dividing line. Thus, M is to be divided by V, giving us the following formula for density.
D = M ÷ V
Formula for Mass

Likewise, to calculate the mass of an object, cover the ‘M’, as shown in the image. The remaining part of it shows D and V adjacent to each other. This indicates that D has to be multiplied by V, giving us the following equation for the mass of an object.
M = D × V
Formula for Volume

To work out the formula for the volume of an object, cover the ‘V’ in the density triangle. What remains is M and D, with M above and D below, separated by a horizontal dividing line. Thus, we get the following formula for the volume of an object.
V = M ÷ D
Example Word Problems
Look at the Chinese proverb in the previous section again. The last part says, ‘I do and I understand’. This is very important, because unless you bring the use of the density triangle into practice, you won’t be able to fully understand how to use it. To help you in this endeavor, in the following lines, we present to you four fully-solved examples. It is advised that you try to solve them on your own before going through the solutions, to get a better grip on this topic.
Example 1: A solid cube has one side equal to 10 cm and a mass equal to 2 kg. Find its density.
Solution: Draw the density triangle as shown in the above section. Now, since we are required to find the density of the cube, we will cover the D part of the triangle, to obtain the following formula,
D = M ÷ V.
Notice that, in this example, the side is given in centimeters. To calculate in S.I. units, it must be converted to meters.
1 m = 100 cm
So, 1 cm = (1 ÷ 100) m
Therefore, 10 cm = (10 ÷ 100) = 0.1 m
Now, the volume of a cube is the cube of its sides. Therefore, V= (0.1)3
V = 0.001 m3
Given mass, M = 2 kg
Putting these values in the above equation for density, we get,
D = (2 ÷ 0.001)
= 200 kg/m3
Example 2: Work out the mass of 300 mL of a solution, if its density is 0.878 gm/mL.
Solution: Here, we are required to find the mass, hence, drawing the density triangle and covering ‘M’ in it, we get,
M = D × V
Now, the given volume (V) = 300 mL. And the given density is 0.878 gm/mL
Since both have the same convention of units, conversion to S.I. units is not needed. Substituting them directly in the equation above,
M = 300 × 0.878
= 263.4 gm.
Example 3: In a cup are present a number of gold-colored beads having a total mass of 500 gm. Through the Archimedes water displacement method, the volume of the beads is found out to be 53.19 cm3. Using the following chart of densities of different metals, can you identify which metal the beads are made up of?
Density of Gold = 19.3 gm/mL
Density of Copper = 8.86 gm/mL
Density of Bronze = 9.87 gm/mL
Solution: We need to identify the metal of which the gold-colored beads are made up of. We know the mass of the beads as well as their overall volume. So, we can find their density using the density triangle.
To calculate density, draw the density triangle and cover the ‘D’ in it. Thus, we obtain the formula for calculating density as,
D = M ÷ V
D = (500 ÷ 53.19)
= 9.87 gm/cm3
Now, we know that 1 cm3 = 1 mL
Thus, we get the density as 9.87 gm/mL. Since this is the density of bronze, the gold-colored beads are actually made up of the metal bronze.
Example 4: Find out the volume of an object made out of silver, having a mass of 3,000 gm, if the density of silver is 10.5 gm/cm3
Solution: To calculate the volume of the object, we draw the density triangle and cover the ‘V’ part of it. The remaining part gives us the following formula,
V = M ÷ D
V = (3,000 ÷ 10.5)
V = 285.71 cm3
Thus, the density triangle can be used to easily and correctly remember the equations for calculating the density, mass, and volume of an object. Once you are clear with the concept, and have tried your hand at the word problems given above, you should become fairly conversant with its use in practical calculations.